10.1 Wave Basics

Waves generally begin as a disturbance of some kind, and the energy of that disturbance gets propagated in the form of waves. We are most familiar with the kind of waves that break on shore, or rock a boat at sea, but there are many other types of waves that are important to oceanography:

• Internal waves form at the boundaries of water masses of different densities (i.e. at a pycnocline), and propagate at depth. These generally move more slowly than surface waves, and can be much larger, with heights exceeding 100 m. However, the height of the deep wave would be unnoticeable at the surface.
• Tidal waves are due to the movement of the tides. What we think of as tides are basically enormously long waves with a wavelength that may span half the globe (see section 11.1). Tidal waves are not related to tsunamis, so don’t confuse the two.
• Tsunamis are large waves created as a result of earthquakes or other seismic disturbances. They are also called seismic sea waves (section 10.4).
• Splash waves are formed when something falls into the ocean and creates a splash. The giant wave in Lituya Bay that was described in the introduction to this chapter was a splash wave.
• Atmospheric waves form in the sky at the boundary between air masses of different densities. These often create ripple effects in the clouds (Figure 10.1.1).

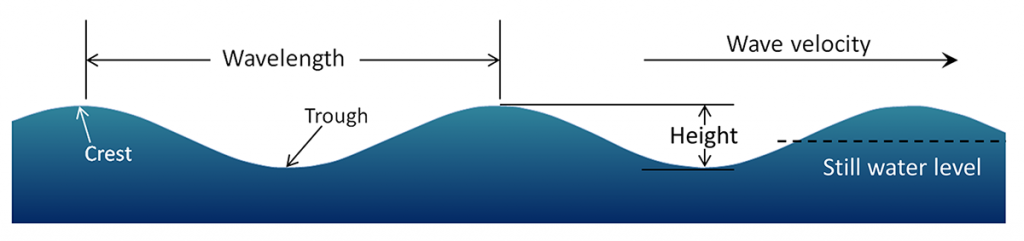
There are several components to a basic wave (Figure 10.1.2):

• Still water level: where the water surface would be if there were no waves present and the sea was completely calm.
• Crest: the highest point of the wave.
• Trough: the lowest point of the wave.
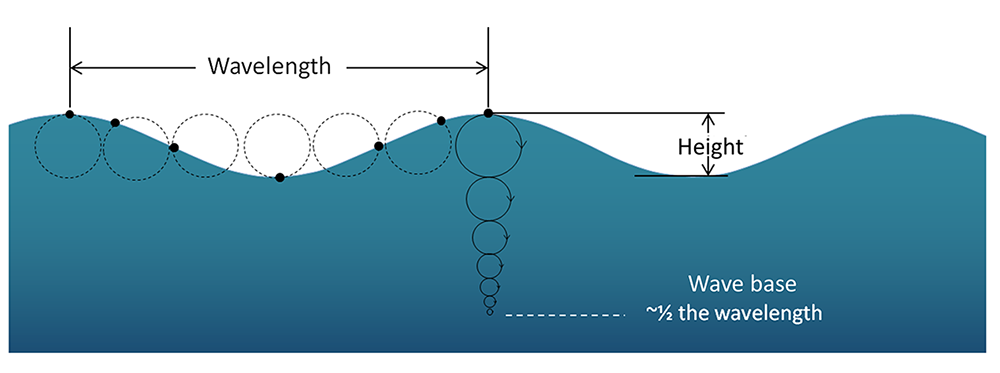
• Wave height: the distance between the crest and the trough.
• Wavelength: the distance between two identical points on successive waves, for example crest to crest, or trough to trough.
• Wave steepness: the ratio of wave height to length (H/L). If this ratio exceeds 1/7 (i.e. height exceeds 1/7 of the wavelength) the wave gets too steep, and will break.

There are also a number of terms used to describe wave motion:

• Period: the time it takes for two successive crests to pass a given point.
• Frequency: the number of waves passing a point in a given amount of time, usually expressed as waves per second. This is the inverse of the period.
• Speed: how fast the wave travels, or the distance traveled per unit of time. This is also called celerity (c), where

c = wavelength  x  frequency

Therefore, the longer the wavelength, the faster the wave.

Although waves can travel over great distances, the water itself shows little horizontal movement; it is the energy of the wave that is being transmitted, not the water. Instead, the water particles move in circular orbits, with the size of the orbit equal to the wave height (Figure 10.1.3). This orbital motion occurs because water waves contain components of both longitudinal (side to side) and transverse (up and down) waves, leading to circular motion. As a wave passes, water moves forwards and up over the wave crests, then down and backwards into the troughs, so there is little horizontal movement. This is evident if you have ever watched an object such as a seabird floating at the surface. The bird bobs up and down as the wave pass underneath it; it does not get carried horizontally by a single wave crest.

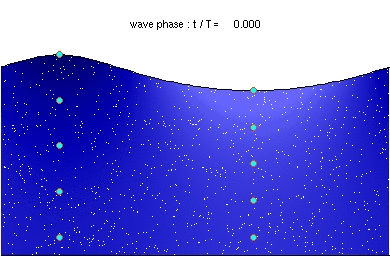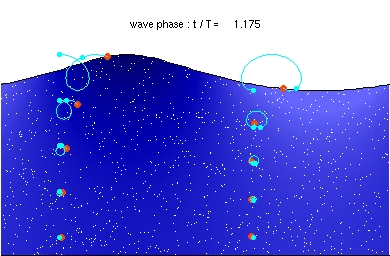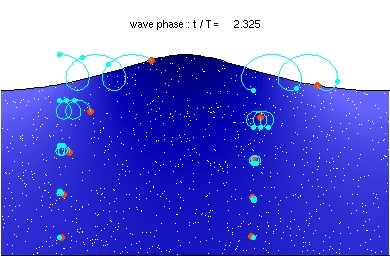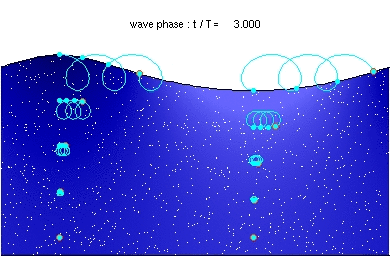
The circular orbital motion declines with depth as the wave has less impact on deeper water and the diameter of the circles is reduced. Eventually at some depth there is no more circular movement and the water is unaffected by surface wave action. This depth is the wave base and is equivalent to half of the wavelength (Figure 10.1.4). Since most ocean waves have wavelengths of less than a few hundred meters, most of the deeper ocean is unaffected by surface waves, so even in the strongest storms marine life or submarines can avoid heavy waves by submerging below the wave base.

When the water below a wave is deeper than the wave base (deeper than half of the wavelength), those waves are called deep water waves. Most open ocean waves are deep water waves. Since the water is deeper than the wave base, deep water waves experience no interference from the bottom, so their speed only depends on the wavelength:

where g is gravity and L is wavelength in meters. Since g and π are constants, this can be simplified to:

Shallow water waves occur when the depth is less than 1/20 of the wavelength. In these cases, the wave is said to “touch bottom” because the depth is shallower than the wave base so the orbital motion is affected by the seafloor. Due to the shallow depth, the orbits are flattened, and eventually the water movement becomes horizontal rather than circular just above the bottom. The speed of shallow water waves depends only on the depth:

where g is gravity and d is depth in meters. This can be simplified to:

Intermediate or transitional waves are found in depths between ½ and 1/20 of the wavelength. Their behavior is a bit more complex, as their speed is influenced by both wavelength and depth. The speed of an intermediate wave is calculated as:

which contains both depth and wavelength variables.